Lycee Francais SAMI Maths Club

The first question of today is how many sides a piece of paper has. So the answer is 2 of course. Now we want to find a way of folding the paper in such a way that there is only one side. One way to verify this is to take a pen and colour the folded piece of paper. If you do not need to turn the piece of paper around, there is only one side. The method to do this is to twist the piece of paper by half a turn and you will obtain a möbius band.

This is all explained in this presentation from Suffolk maths, that also contains a brilliant exploration table. Your challenge is to fill in the first three lines and to come up with three other results to add to the table below.

Good luck!

This week we worked through another brilliant puzzle from the Math Pickle team called McGuire the Gathering.

Lots of fun trying to solve both on pen and paper and with excel!

We used this website to solve a series of challenges where you start with front, side and top elevations to build the full picture of 3D shape – building up to using only two views and silhouettes.

In pairs we played the following game a couple of times.

One person is heads (player 1), the other person is tails (player 2). Toss the coin a maximum of 10 times, player 1 gets a point if the coin lands on heads, and player 2 gets a point if the coin lands on tails. If one person becomes three points ahead of the other the game stops and they win. If no one is 3 points ahead after ten tosses the game is a tie.

Which of the following scores are impossible in this game and why? 5-2, 7-4, 6-2

Think of ways the game could end in a tie.

What is the chance of winning this game?

This following table will help.

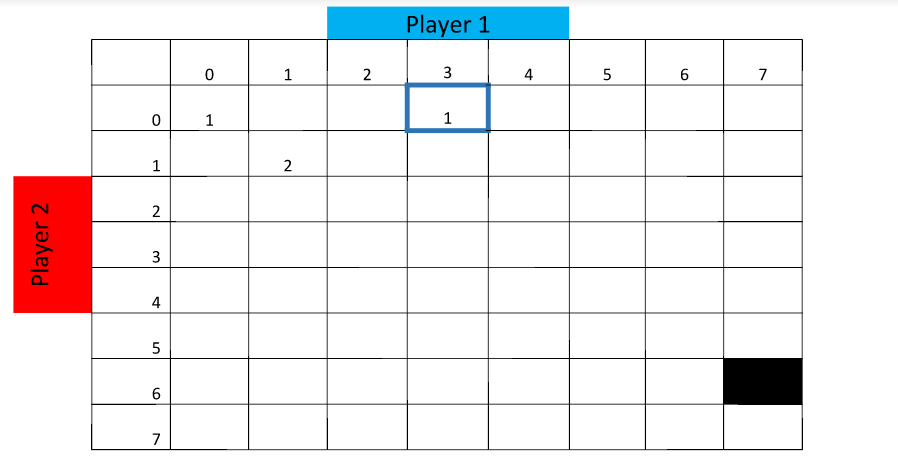
Fill in the table shading out impossible final scores, highlighting winning final scores for Player 1 and Player 2, and highlighting final scores which indicate a draw.
Then write in each box how many ways there are to end up at each score.

A few boxes have been filled in – for example 3-0 is a winning score for player 1, and there is only 1 way to get to that score (1-0 then 2-0 then 3-0). There are two ways to get to a score of 1-1 (0-1 then 1-1 or 1-0 then 1-1). There is a 1 in the 0-0 box as there is just one way to start the game.

See  here for the solution, contained in an excellent presentation by Alex in the Further Maths class in PAL. This puzzle is the starting point for analysing how likely you are to win a tennis match if you only have a one third chance of winning each point. The idea and diagrams in the presentation were taken from the book Game, Set and Math by Ian Stewart.

Unit fractions are fractions that are written in the form ¹⁄_n.

Today’s challenge is to find sums of different unit fractions that are equal to another unit fraction.

For example: ¹⁄₂ = ¹⁄₃ + ¹⁄₆

Let’s see if there is a rule: which of the following are right and which are wrong?

¹⁄₂ = ¹⁄₁₀ + ¹⁄₂₀

¹⁄₃ = ¹⁄₄ + ¹⁄₁₂

¹⁄₃ = ¹⁄₇ + ¹⁄₂₁

¹⁄₄ = ¹⁄₅ + ¹⁄₂₀

The next challenge is to spot the pattern in these sums of unit fractions:

¹⁄₆ = ¹⁄₇ + ¹⁄₄₂

¹⁄₆ = ¹⁄₈ + ¹⁄₂₄

¹⁄₆ = ¹⁄₉ + ¹⁄₁₈

¹⁄₆ = ¹⁄₁₀ + ¹⁄₁₅

Bear in mind that ¹⁄₆ = ¹⁄₁₂ + ¹⁄₁₂ is wrong because both unit fractions are the same.

Try and use this to example to find all the unit fraction sums that add up to ¹⁄₁₈.

What if original fraction is not a unit fraction?

Egyptians had a tendency to write fractions as sums of unit fractions.

Of course, there is an infinite number of ways to do this. Let’s take  for example.

²⁄₃ = ¹⁄₃ + ¹⁄₄ + ¹⁄₁₂

²⁄₃ = ¹⁄₃ + ¹⁄₅ + ¹⁄₂₀ + ¹⁄₁₂

²⁄₃ = ¹⁄₄ + ¹⁄₁₂ + ¹⁄₇ + ¹⁄₄₂ + ¹⁄₃₁ + ¹⁄₉₃₀ + ¹⁄₂₁ + ¹⁄₄₂₀ + ¹⁄₁₃ + ¹⁄₁₅₆

Etc.

But how about expressing non unit fractions as sums of two unit fractions? Here is one example:

²⁄₃ = ¹⁄₂ + ¹⁄₆

But can all fractions with numerator 2 be written as the sum of just 2 unit fractions? Can you prove it?

Let’s finish off with the greedy algorithm. This algorithm, which was developed by Fibonacci, allows you to quickly find a non-unit fraction as the sum of several unit fractions.

For example, let’s take ¹¹⁄₁₂. The first step is to find the largest unit fraction below the other fraction. In this case, that fraction is ¹⁄₂. Then, you should subtract ¹⁄₂from ¹¹⁄₁₂, which gives ⁵⁄₁₂. This means that ¹¹⁄₁₂ = ¹⁄₂ + ⁵⁄₁₂ .  Repeat this with ⁵⁄₁₂ and you should get:

¹¹⁄₁₂ = ¹⁄₂ + ¹⁄₃ + ¹⁄₁₂

If you would like to learn more, you can to the NRICH website with these links:

https://nrich.maths.org/6540

https://nrich.maths.org/1173

https://nrich.maths.org/6541

This week we looked at a nice activity exploring the possibility (or not!) or tiling (covering) various shapes using the tiles above.

Here is one interesting one to try:

Here you can find a worksheet to try out and here are some notes, solutions and further reading, all courtesy of Balázs Szendrői.

The challenge this week is to switch round the four knights as shown above, using only regular knights chess move (L shape – one along two up/down, or two along one up/down). Two knights can’t occupy the same space so you will need to be careful!

For an interesting discussion of the solution see this video .

Here is probably the hardest puzzle we’ve looked at so far in maths club …

Two perfect logicians, Sam and Polly, are told that integers x and y have been chosen such that 1 < x < y and x+y < 100.  Sam is given the value x+y and Polly is given the value xy.

They then have the following conversation.

Polly:  I cannot determine the two numbers.

Sam:  I knew that.

Polly:  Now I can determine them.

Sam:  So can I.

Given that the above statements are true, what are the two numbers?

Starting points:

Before Polly and Sam say anything:

What is the range of numbers Sam could be given?

What is the range of numbers Polly could be given?

What special type of number can the product of x and y never be?

What about the square of these numbers?

After Polly’s first statement:

Give two examples of products that Polly can not be given.

After Sam’s first statement:

 Give two examples of sum’s that Sam can not be given.

Next steps …

At this point you probably want to start using a computer to generate a list of numbers that they could be given.

This puzzle comes from Alex Bellos’ column in the Guardian. It has been adapted from one by Prem Prakash, an electrical engineer from Bangalore, India, who has taken early retirement to develop puzzle-based teaching workshops.

You and your two friends Pip and Blossom are captured by an evil gang of logicians. In order to gain your freedom, the gang’s chief, Kurt, sets you this fearsome challenge.

The three of you are put in adjacent cells. In each cell is a quantity of apples. Each of you can count the number of apples in your own cell, but not in anyone else’s. You are told that each cell has at least one apple, and at most nine apples, and no two cells have the same number of apples.

The rules of the challenge are as follows: The three of you will ask Kurt a single question each, which he will answer truthfully ‘Yes’ or ‘No’. Every one hears the questions and the answers. He will free you only if one of you tells him the total number of apples in all the cells.

Pip: Is the total an even number?

Kurt: No.

Blossom: Is the total a prime number?

Kurt: No

You have five apples in your cell. What question will you ask?

See here for the solution.

Today we looked at how we can transform a blank sheet of A4 paper into many different shapes such as an equilateral triangle and a kite. To do so you can either try to figure it out yourself or you can follow the Origami instructions for each different shapes.

If you have anymore interesting ideas please share them in the comment section below and if you want an extra challenge try proving that all of these folds actually give regular shapes bearing in mind the fact that the ratio of the sides in an A4 paper is 1:root 2