
Today we worked on this fun puzzle from Mathpickle.com.
You can work through the website at your own pace, but make sure you don’t read beyond the slide below until you have tried it yourself (Spoiler alert!).


Today we worked on this fun puzzle from Mathpickle.com.
You can work through the website at your own pace, but make sure you don’t read beyond the slide below until you have tried it yourself (Spoiler alert!).

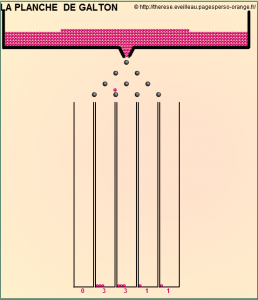
This week we looked at an online simulation of the Galton board (or Bean Machine), which is a device where beads are dropped from a funnel at the top through ranks of nails.
Each time a bead strikes a nail it has a 50% chance to veer left and a 50% chance to veer right.
Each bead eventually drops in one of the column A, B, C, D or E.
1. Do you think the probabilities for a bead to land in A,B,C,D or E are equal ?
2. If not which column has the highest probability ?
3. Can you do a computer simulation of 10,000 beads dropping in a Galton
board using scratch or Python ?
When you throw two dice and add the numbers together what number are you most likely to get? What is the smallest number you can get? What is the biggest? Are all numbers equally likely?
We used python to simulate rolling a dice 100 times and plotted the results using pygal. Step by step instructions are here.
A big challenge would be to program the following game on python:
The game of Pig is a two player game played with two six-sided dice. The object of the game is to reach 100 points of more. Play is taken in turns. On each person’s turn that person has the option of either:

We really enjoyed working on the following puzzle from Nrich.
Thirteen nations competed in a sports tournament. Unfortunately, we do not have the final medal table, but we have the following pieces of information:
1. Turkey and Mexico both finished above Italy and New Zealand.
2. Portugal finished above Venezuela, Mexico, Spain and Romania.
3. Romania finished below Algeria, Greece, Spain and Serbia.
4. Serbia finished above Turkey and Portugal, both of whom finished below Algeria and Russia.
5. Russia finished above France and Algeria.
6. Algeria finished below France but above Serbia and Spain.
7. Italy finished below Greece and Venezuela, but above New Zealand.
8. Venezuela finished above New Zealand but below Greece.
9. Greece finished below Turkey, who came below France.
10. Portugal finished below Greece and France.
11. France finished above Serbia, who came above Mexico.
12. Venezuela finished below Mexico, and New Zealand came above Spain.
We came up with different strategies to sort out the medal table, and we were largely successful eventually, but we were all impressed by a quick way to solve it!

We played a two player strategy game today, using all the information from +plus magazine.
The game is played as follows. At the start of a game each player decides on their three colour sequence for the whole game. The cards are then turned over one at a time and placed in a line, until one of the chosen triples appears. The winning player takes the upturned cards, having won that “trick”. The game continues with the rest of the unused cards, with players collecting tricks as their triples come up, until all the cards in the pack have been used. The winner of the game is the player that has won the most tricks. An average game will consist of around 7 “tricks”.
e.g. Player 1 picks RRB, Player 2 picks RBB and see who wins.
The question we thought about was:
There is a related game called Penny’s game, using Heads and Tails of a coin toss instead of playing cards. In this version, you just play until one person has won a “trick”. We worked out some of the odds given in the first table in this article.
We ended up having to sum a geometric series! Great fun!
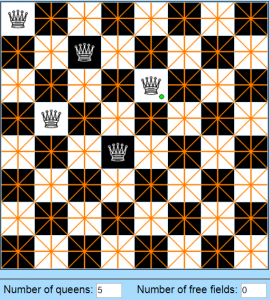
Welcome back everyone!
Our first puzzle this year was to place 8 queens on a chessboard such that none of the queens could take any of the others. Above is a fail – only 5 queens are on the board and they are no places left to put any more.
Here is H.B.Meyer’s website – try for yourself to fit 8 queens on the board, it is possible!
We then looked at changing the size of the board from an 8 by 8 to smaller sizes, e.g. can you fit 5 queens on a 5 by 5 board? Here is a place to try this. And if so, how many “unique” solutions are there? We defined a unique solution as being one that did not look like any others we had found when we rotated our paper or put it up to the light so it appeared flipped!
There is a great numberphile video on this puzzle, and all the answers for different size boards on wikipedia.
On a piece of paper, draw a triangle (any triangle -right angled, equilateral, isosceles, scalene). Colour one of the vertices red, the second blue, and the third green.
Next, take three dice – one red, one blue, and one green.
Now draw a point in the triangle. This point is the seed for the game. Then roll the die. Whichever die shows the highest number (throw again if there is a tie for the highest), draw a point halfway inbetween the seed and the appropriately colored vertex. Now do the same, using your new point as the seed for the next. After a few rolls you might have a drawing like this:
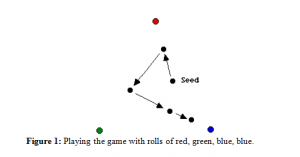
Now continue in this fashion for five rolls of the dice. Then rub out all the points except the most recent seed and the coloured points.
Now carry on but don’t erase any points.
Can you guess what pattern your points are going to make? You might be surprised!
You might want to use technology to help you find out. Here are some instructions for using geogebra.

The aim of this puzzle is to switch the red frogs with the blue frogs according to the following rules:
How many moves does this puzzle take?
How about if we change the number of frogs? Can you find a rule for the number of moves it will take based on how many frogs there are?
NRich has an applet with nice graphics here, but you are allowed to go backwards in their version, so it is is best to use this one to help you count the moves.
For interested facilitators extra notes and the solutions are here.

Today we solved an intriguing homework riddle with the help of a Venn diagram. We had clues such as
“Seven students in total have Maths homework but Miya isn’t one of them.”
and
“Rory has more homework than Aidan.”
Using all the clues, which you can find here, we were able to work out which students had which pieces of homework.
It was a nice challenge! The solution can be found here. This activity was originally found on the TES website.
We spent this maths club playing the highly addictive game Square it from Nrich.