Today we tried a lovely puzzle from Alex Bellos’ column in the guardian.
The interactive app on Scratch was very helpful.
Today we tried a lovely puzzle from Alex Bellos’ column in the guardian.
The interactive app on Scratch was very helpful.
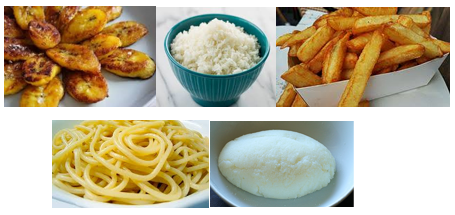
3 sisters can never choose what to have for dinner. So they take a vote. 1 is their first choice, 5 is their least favourite. What should they have for dinner?
3 filles n’arrivent jamais à choisir quoi manger pour le dîner. Elles décident donc de faire un vote. 1 est leur premier choix, 5 leur dernier. Que devraient-elles manger pour le dîner ?
Rosie
| Food/Nourriture | Preference/Préference |
| Plaintain | 1 |
| Rice | 2 |
| Chips | 4 |
| Spaghetti | 5 |
| Ugali | 3 |
Precious
| Food/Nourriture | Preference/Préference |
| Plaintain | 5 |
| Rice | 2 |
| Chips | 3 |
| Spaghetti | 4 |
| Ugali | 1 |
Florence
| Food/Nourriture | Preference/Préference |
| Plaintain | 2 |
| Rice | 3 |
| Chips | 1 |
| Spaghetti | 4 |
| Ugali | 5 |
Which dish should their mother cook?
Quel plat devrait cuisiner leur mère ?
Can you make an argument for each of the dishes?
Peux-tu donner des arguments pour défendre chaque plat ?
Give us your suggestions in the comments section!
Donnes-nous tes suggestions dans les commentaires !
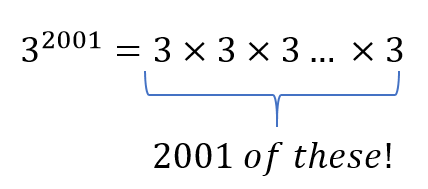
3 to the power of 2001 is a very big number (954 digits long!!). A regular calculator will not be able to calculate it.
Without calculating the value of this number, can you find the remainder when it is divided by 7?
For more explanation see the English and French versions of the puzzle.
And here are the solutions in English and French.
This problem was posted originally on the Nrich website .

The rugby world cup is up and running. If you walked in half way through a game and saw that Scotland had 21 points (if only!), you would not know whether they had scored 7 penalties or 3 converted tries.
What is the highest score that you could know exactly how the points have been scored?
For more explanation see the English and French versions of the puzzle.
And the solutions in English and French.
.
We found this puzzle in Alex Bellos’ great column in the guardian.
Student versions of todays puzzle: English Français
Facilitator versions of todays puzzle: English Français
Konstantin Oskolkov of the Steklov Mathematical Institute in Moscow was told about this puzzle by a stranger c.1980. It has become known as Bulgarian Solitaire. It has been written up by MathPickle in terms of King Kong here.

Today we revisited an intriguing investigation of a simple recurrence relation.

We really enjoyed working on this puzzle from Nrich today.
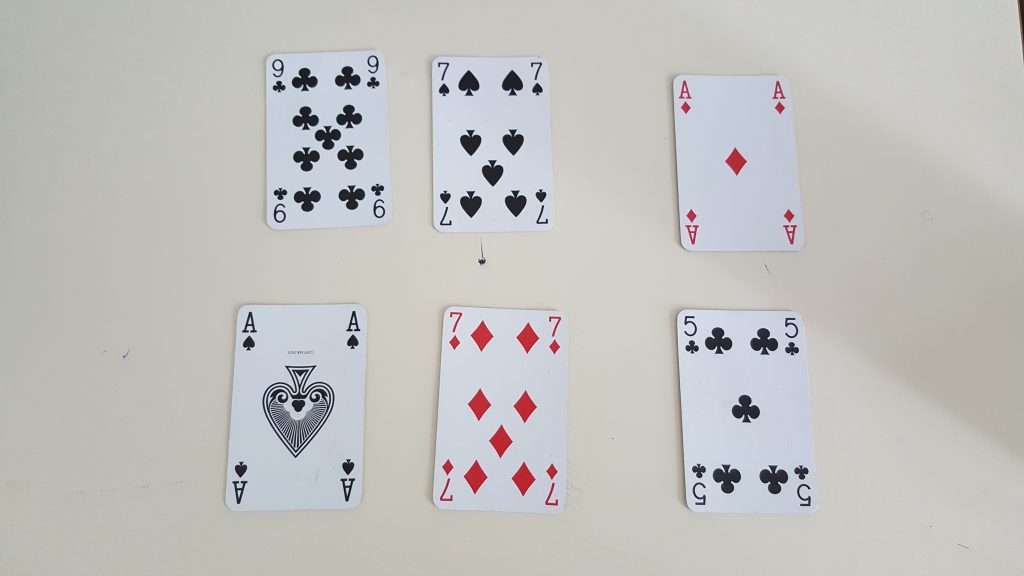
Today we did one of the 52 activities in mathemagician Andrew Jeffrey’s great book. Andrew very kindly donated the proceeds of this book to SAMI.
This activity involved shuffling the Aces through to 9s in a pack of cards and setting them out in the following format to gain the biggest total. The first two cards represent a two digit number. We played many games of 1 min each to see who could find the biggest total (without a calculator!) and then enjoyed discussing strategy until we found (we think!) a perfect strategy.
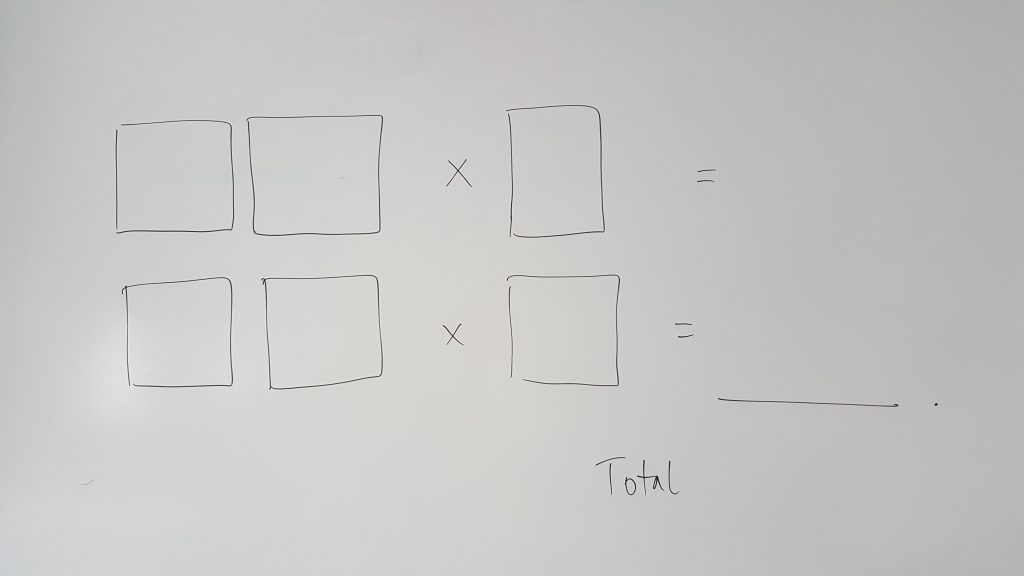
The total for the layout of the cards at the top would be 97×1+17*5=182. Can you do better with those six cards?

We played a really fun version of Nim today. The online version seems to work best with two players. We found the computer only chooses to mark one dot each time … which is not a winning strategy!
Today we revisted one of our favourite puzzles.