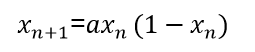Unit fractions are fractions that are written in the form 1⁄n.
Today’s challenge is to find sums of different unit fractions that are equal to another unit fraction.
For example: 1⁄2 = 1⁄3 + 1⁄6
Let’s see if there is a rule: which of the following are right and which are wrong?
1⁄2 = 1⁄10 + 1⁄20
1⁄3 = 1⁄4 + 1⁄12
1⁄3 = 1⁄7 + 1⁄21
1⁄4 = 1⁄5 + 1⁄20
The next challenge is to spot the pattern in these sums of unit fractions:
1⁄6 = 1⁄7 + 1⁄42
1⁄6 = 1⁄8 + 1⁄24
1⁄6 = 1⁄9 + 1⁄18
1⁄6 = 1⁄10 + 1⁄15
Bear in mind that 1⁄6 = 1⁄12 + 1⁄12 is wrong because both unit fractions are the same.
Try and use this to example to find all the unit fraction sums that add up to 1⁄18.
What if original fraction is not a unit fraction?
Egyptians had a tendency to write fractions as sums of unit fractions.
Of course, there is an infinite number of ways to do this. Let’s take for example.
2⁄3 = 1⁄3 + 1⁄4 + 1⁄12
2⁄3 = 1⁄3 + 1⁄5 + 1⁄20 + 1⁄12
2⁄3 = 1⁄4 + 1⁄12 + 1⁄7 + 1⁄42 + 1⁄31 + 1⁄930 + 1⁄21 + 1⁄420 + 1⁄13 + 1⁄156
Etc.
But how about expressing non unit fractions as sums of two unit fractions? Here is one example:
2⁄3 = 1⁄2 + 1⁄6
But can all fractions with numerator 2 be written as the sum of just 2 unit fractions? Can you prove it?
Let’s finish off with the greedy algorithm. This algorithm, which was developed by Fibonacci, allows you to quickly find a non-unit fraction as the sum of several unit fractions.
For example, let’s take 11⁄12. The first step is to find the largest unit fraction below the other fraction. In this case, that fraction is 1⁄2. Then, you should subtract 1⁄2from 11⁄12, which gives 5⁄12. This means that 11⁄12 = 1⁄2 + 5⁄12 . Repeat this with 5⁄12 and you should get:
11⁄12 = 1⁄2 + 1⁄3 + 1⁄12
If you would like to learn more, you can to the NRICH website with these links:
https://nrich.maths.org/6540
https://nrich.maths.org/1173
https://nrich.maths.org/6541
![]()