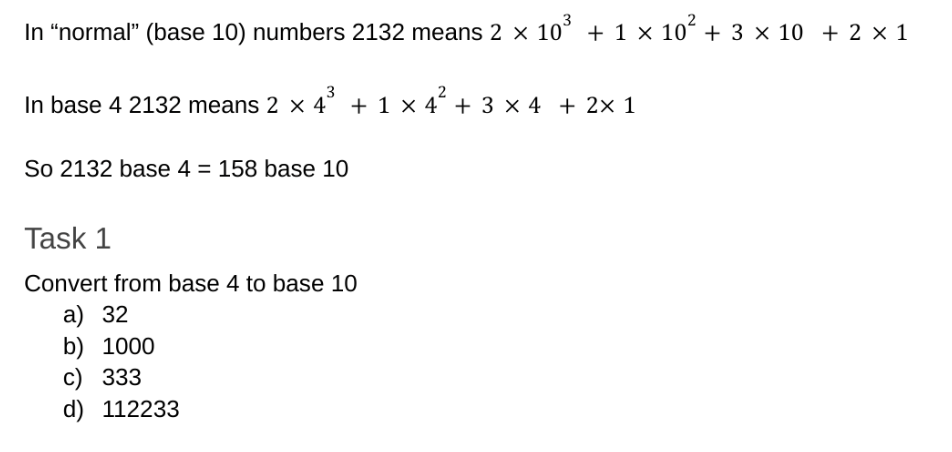This activity comes from the great website mathsisfun.com.
Have you ever coloured in a pattern and wondered how many colours you need to use?
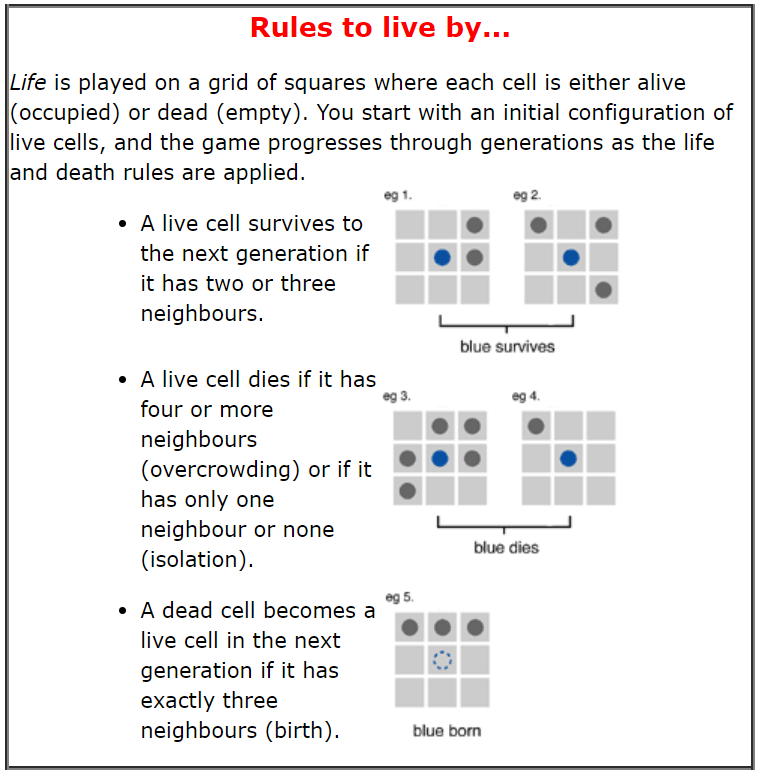
There is only one rule
Two sections that share a common edge cannot be colored the same!
Having a common corner is OK, just not an edge.
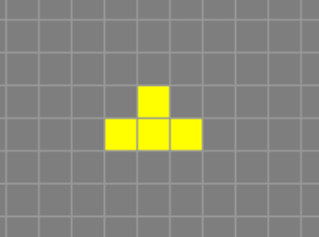
Let’s start with a simple pattern like a group of nine squares:
What is the minimum colours you need to colour the pattern of nine squares?
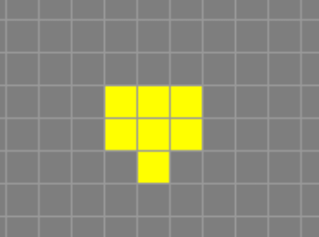
A Little More Complicated
How about this one?
How many colours do you need this time?
Even More Complicated
Let’s try another:
How many colours do you need this time?
Nine? Eight? Seven? Six? Five? Four?
Maps
Things get more interesting if we want to colour a map.
Here is a map of Africa, showing six countries and how they border on each other:
Try colouring in the map and see what is the fewest number of colours you need.
Extension
Can you draw a map with 3 countries such that every country has exactly two neighbours, and then colour it.
Can you draw a map with 4 countries such that every country has exactly two neighbours, and then colour it.
Can you draw a map with 6 countries such that every country has exactly four neighbours, and then colour it.
Can you draw a map with 12 countries such that every country has exactly five neighbours, and then colour it.
Further Reading
The proof of the four colour theorem was famously tricky, and comes from graph theory, where mathematicians investigate an equivalent problem of colouring vertices of a network so that no edge has endpoints the same colour. The original four-colour proof was attempted by Alfred Kempe in 1879, but unfortunately Percy John Heawood found an error 11 years later. However his work was not useless, as Percy was able to prove the five-colour theorem (that one can colour a map with no two adjacent regions the sample colour using at most 5 colours) based on Kempe’s work. The four colour theorem was finally proved in 1976 by Kenneth Appel, Wolfgang Haken, and John Koch using a computer to check it. This was the first major theorem to be proved using a computer. They checked around 1500 configurations using about 1200 hours of computer time. Some people were sceptical about a proof using a computer but independent verification soon convinced everyone that the four colour theorem had finally been proved.
You can read more here.