This page is intended to help students studying the French Baccalauréat who wish to go to a British University to study a maths related course.
Here is the specification for A-level mathematics. What follows is links to resources to help fill the gaps. With special thanks to Dr Frost for making his powerpoints freely available.

Proof is a stand alone topic in the British curriculum. Here is a powerpoint and some questions to try can be found here.

For example, you should be able to calculate the following in your head:
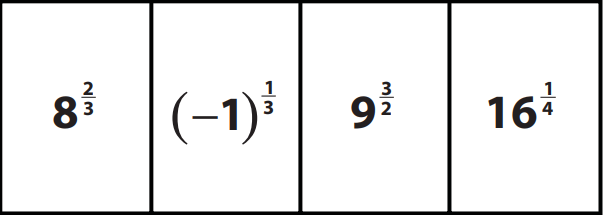
Here is an explanation of rational exponents and here are some exercises to try. Some good questions can be found in this worksheet (answers).
In the British curriculum it is expected that an expression such as x^2+5x+6 for example can be factorised into (x+3)(x+2). This is without using a calculator and without finding the solutions to
x^2+5x+6 =0, simply by thinking of two numbers that multiply to 6 but add up to 5.
This is also expected for equations such as :

You could realise that it would have to be ( – 1)( – 1) and then guess (3x – 1)(3x – 1) or (9x – 1)(x – 1) and see which worked. For other tips and tricks see here, and here are some questions to try.

You may be used to set notation, but perhaps not in this context. All that is expected of you is that you can write the answer to quadratic inequalities in this way.