The lowest common multiple of 6, 14, and 60 is 420 (7×6×10). So 7 hours after noon.
7pm is the answer.
The lowest common multiple of 6, 14, and 60 is 420 (7×6×10). So 7 hours after noon.
7pm is the answer.
Matt, Noah, Olivia and Penny are at the Christmas market.
A Christmas tree seller is selling decorated and undecorated trees.
There is one more decorated tree than undecorated tree.

Matt says that the number of decorated trees is prime.
Noah says the total amount of trees is more than 8 and less than 12.
Olivia says that there are 4 undecorated trees.
Penny says that Olivia or Noah are lying.
Only one of the four are lying.
How many trees are there?
Solution is here.
There must be 4 decorated trees and 5 not decorated.
The first assumption that can be made is that either Penny, Olivia or Noah are lying, because if Penny is lying, everyone else is truthful, and if Matt is lying, Penny states that Noah or Olivia are also lying, so either one of those three are lying, which means there are too many liars.
Therefore, we have two truths: there is one more decorated tree than undecorated, and the number of trees is prime, what Matt says. Starting with all primes smaller than ten, there could be 2 undecorated and 3 decorated trees, 4 undecorated and 5 decorated trees, or 6 undecorated and 7 decorated trees.
If Olivia is telling the truth, the total is 9 (4+5) , which also means that Noah is telling the truth ( 8 < 9 < 12), and Penny is lying. In the other cases, both Noah and Olivia must be lying at once(2+3 < 8 and 6+7 > 12, none of these possibilites contain 4).
The only possible solution is when Penny is lying, and we’ve seen that in this scenario, there are 5 decorated trees and 4 undecorated trees.
You could solve this by simultaneous equations, but there is a quick way to do it without working out how much each present costs.
Add together all the amounts – this will give you double the cost as each colour present appears twice. Then just divide your answer by 2.
(£22+£15+£16+£32+£37)/2 = £61
 Five presents were bought for Christmas
Five presents were bought for Christmas
The red and purple presents together cost £22
The purple and blue presents together cost £15
The blue and gold presents together cost £16
The gold and green presents together cost £32
The green and red presents together cost £37
What is the total cost of all 5 presents?
Solution is here.
The possibilities are:
(Wins, draws, goals)
Rudolphs: (0, 0, 8) or (0, 1, 3)
Comets: (0, 0, 14) or (0, 1, 9) or (0, 2, 4) or (1, 0, 4)
Vixens: (0, 0, 9) or (0, 1, 4)
If there were 3 games, then there would be 30 points
for wins and draws, leaving just 1 point for goals,
so that is not possible, since each team scored in each game.
Thus, there were only 2 games,
yielding 20 points for wins and draws, 11 for goals,
which reduces the possibilities to
Rudolphs: (0, 1, 3)
Comets: (0, 2, 4) or (1, 0, 4)
Vixens: (0, 1, 4)
Rudolphs had a draw (and maybe a loss).
Comets had 2 draws or a win and no other match.
Vixens had a draw (and maybe a loss)
If Comets had 2 draws, then both games were draws,
but that is not possible since total goals was 11, which is odd.
So Comets had a win against one of the others,
and the other game was a draw between the Rudolphs and the Vixens.
The score of Rudolphs-Vixens draw then was either 1-1, 2-2, or 3-3
Since Vixens scored more goals than Rudolphs, they
are the ones who lost to Comets, and Rudolphs, only playing once,
scored all 3 goals in that game, equalling Vixens.
Comets scored all 4 of their goals in their only game, where
Vixens scored one additional goal.
The results were:
Comets 4, Vixens 1
Rudolphs 3, Vixens 3
Snowman had never fully understood the points system in football, and felt that the scoring of goals should be encouraged. His idea is that 10 points should be awarded for a win, 5 points for a draw and 1 point for each goal scored, whatever the result of the match.
This was tried with three teams: Rudolph, Comet and Vixen.
Each team scored at least one goal in every match and no team played another more than once.
Rudolph scored 8 points, Comet 14 points and Vixen scored 9 points.
Find the score in each match.
Solution is here.
It can be proven that there are no integer (whole number) solutions to

So there are no integer solutions to
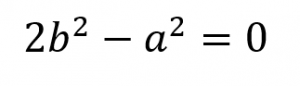
But can you find positive integer values of a and b that nearly work? That give the answer 1 or -1?
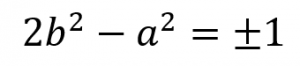
Can you find a pattern to all your solutions?
What does a/b give a good approximation to?

Today we worked on this fun puzzle from Mathpickle.com.
You can work through the website at your own pace, but make sure you don’t read beyond the slide below until you have tried it yourself (Spoiler alert!).

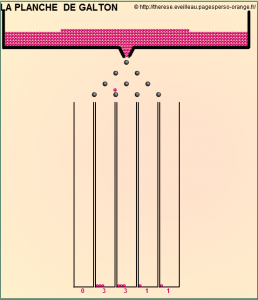
This week we looked at an online simulation of the Galton board (or Bean Machine), which is a device where beads are dropped from a funnel at the top through ranks of nails.
Each time a bead strikes a nail it has a 50% chance to veer left and a 50% chance to veer right.
Each bead eventually drops in one of the column A, B, C, D or E.
1. Do you think the probabilities for a bead to land in A,B,C,D or E are equal ?
2. If not which column has the highest probability ?
3. Can you do a computer simulation of 10,000 beads dropping in a Galton
board using scratch or Python ?