This activity was created using content from this worksheet from Maths Craft NZ and this Mathigon Tesselation Activity.
A tiling consists of covering a surface (a plane) using copies of shapes, called tiles, without overlapping or leaving gaps.
For example if we use a square or an equilateral triangle as a tile we can create a tiling that will cover an infinite plane.
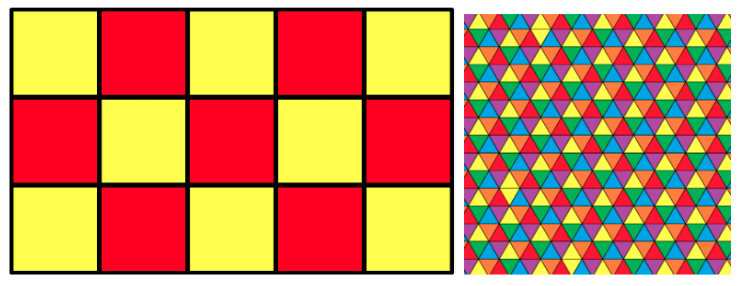
Only one other regular (all sides and angles the same) polygon can tile the plane, which polygon is this?
Can you prove that there are no others?
You can also use multiple shapes to tile the plane. For example:
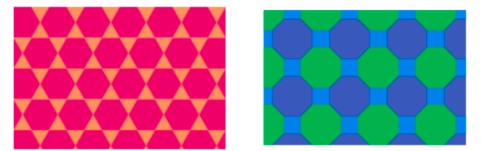
You can also use irregular shapes to tile the plane, any triangle or quadrilateral works:
Exactly 15 different pentagons will work, for example:

Go to Mathigon and make a tiling using either one regular polygon, one irregular triangle or quadrilateral or multiple shapes. Use the “Polygons and Shapes” or Pentagon Tilings” menus. You can change colour at the bottom.
All the above tilings are periodic tilings which means they have translational symmetry.
Aperiodic Tiling
Go to Mathigon and make a tiling using either one regular polygon, one irregular polygon or multiple shapes.Use the “Polygons and Shapes” or Pentagon Tilings” menus. You can change the colour of the tiles at the bottom and you can quickly duplicate whole sections of your tiling by highlighting and clicking on the copy icon.
All the above tilings are periodic tilings which means they have translational symmetry.
From wikipedia:
Covering a flat surface (“the plane”) with some pattern of geometric shapes (“tiles”), with no overlaps or gaps, is called a tiling. The most familiar tilings, such as covering a floor with squares meeting edge-to-edge, are examples of periodic tilings. If a square tiling is shifted by the width of a tile, parallel to the sides of the tile, the result is the same pattern of tiles as before the shift. A shift (formally, a translation) that preserves the tiling in this way is called a period of the tiling. A tiling is called periodic when it has periods that shift the tiling in two different directions.
The tiles in the square tiling have only one shape, and it is common for other tilings to have only a finite number of shapes. These shapes are called prototiles, and a set of prototiles is said to admit a tiling or tile the plane if there is a tiling of the plane using only these shapes. That is, each tile in the tiling must be congruent to one of these prototiles.
A tiling that has no periods is non-periodic. A set of prototiles is said to be aperiodic if all of its tilings are non-periodic, and in this case its tilings are also called aperiodic tilings.
In 1961 Robert Berger constructed such a set, using more than 20,406 tiles. Later he found a much smaller set of 104, and Donald Knuth was able to reduce the number to 92.
Then Raphael M. Robinson found this set of 6 tiles in 1971:
In the mid 70s Roger Penrose found a two tile set that would work. This discovery eventually led to him suing a toilet paper company and to a new way to make non stick frying pans.
And finally in 2022 a retired systems printing engineer found a single tile – called an Einstein tile for the German for one stone.
A Penrose tiling uses only two shapes, with rules on how they are allowed to go together. These shapes are called kites and darts and in the mathigon version they have white lines that need to match up. This means for example that the dart cannot sit on the kite. Three possible ways to start a Penrose tiling are:
These are called the ‘star’, ‘sun’ and ‘ace’ respectively.
Using the pictures above, can you calculate all the angles in the dart and the kite?
There are 4 other ways to arrange the tiles around a point. Can you find them all?
The solution is here
Oxford Online Maths club have done a nice video on this topic. Roger Penrose is a famous professor at Oxford and has a floor built in his honour with his tiles:
Here is an editable version of this page.