
Here is a series of puzzles with red and blue hats that will challenge your logical skills.

Here is a series of puzzles with red and blue hats that will challenge your logical skills.
Today we did one of our favourite puzzles inspired by this Numberphile video by Neil Sloane.
Starting with 2 ones, what is the maximum number you can place on an infinite grid according to the rule:
“You can place a number if all the numbers immediately around it add up to itself”
Here is a way to get up to 4:
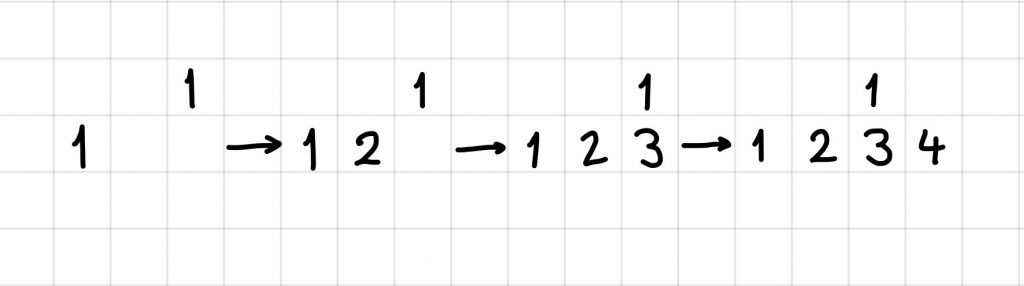
But then we are stuck as there is nowhere to put the 5. Can you do better than 4?
You can choose where to place the 2 ones to start with, for example:


This puzzle can be tried with any number of 1s to start with.
What is the maximum number you can place starting with three 1s?
Some solutions are here.

This investigation is based on the ancient Greek Apollonius Problem: Can you draw a circle that just touches three given circles? How many can you draw?
We tried this by hand and then on Geogebra following these instructions.
The maximum number of circles possible was unsolved for more than 2000 years, but we solved it! For the solutions and more see the links below:

Thanks to Barasa in Kenya for telling us about this one …
Challenge 1
Take 10 cards from a deck – A,2,3, … 10. Ace represents 1.
Arrange the cards in a pile face down so that when you show the first card it is the Ace. Remove this card. Then count to two putting the first card on the bottom of the pile. The second card should be the two. Show it and remove it. Then count to three, each time you say a number you should be putting a card on the bottom and then when you get to 3 you show the 3 and remove it from the pile.
Arrange the cards so that this works for every number from A to 10. By the time you get to 9 you should only have two cards left in your hand! Cycle through them counting 1, 2, 3, … 9 and show the 9. And then you just have 10 left and the trick is over.
Challenge 2
Try to arrange the cards to perform the same trick except you don’t remove the card when you show it. The card is just placed at the bottom of the pile and you start counting the next number.
You should find out that this is impossible for 10 cards. Is there a number of cards that it would work for?
We tried a dice puzzle today set a while back by Matt Parker.
You only have the tool above – three dice inside a cube.
But you want to play a game that requires throwing a single dice and then a game that requires throwing two dice and adding their score together. Is there a way that you can use the sum of these three dice to simulate both of these scenarios?
Task 1
First work out the distribution of numbers you can score with three dice
Can you map this in a nice way to the distribution for 1 dice?
Task 2
How about for 2 dice?

Might not be able to do it using (only) the sum …
Printable worksheet here.
Succinct solution here or watch the full brilliant video by Matt.
Today we worked on an absorbing puzzle that you can play online here:
https://www.cleverlearning.co.uk/blogs/blogConwayInteractive.php
The Irish logarithm is an algorithm invented by Percy Ludgate in 1909 for multiplying single-digit numbers. The idea was to program a computer to do these calculations.
The algorithm uses two tables to perform the multiplication. With just these tables you can calculate products up to 9×9 simply by adding two numbers together.
Here are blank versions of the two tables below (and as a pdf).
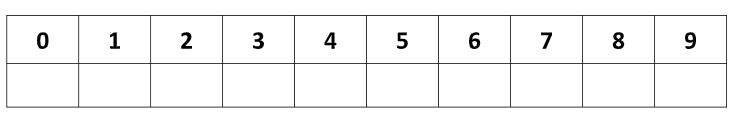
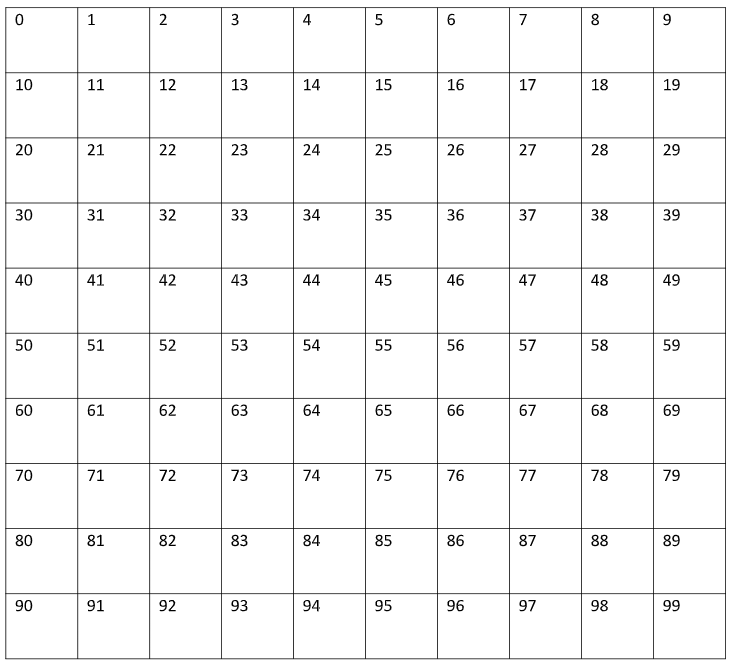
Our challenge was to fill in these tables. Here is the start of an attempt that would not work …
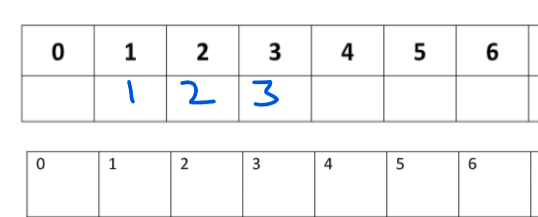
1 x 2 would be 1+2 so the correct answer of 2 needs to be in the box called 3.
1×3 would be 1+3 so the correct answer of 3 needs to be in the box called 4.
2×3 would be 2+3 so the correct answer of 6 needs to be in the box called 5.
So far so good ….

But … 2×2 would be 2+2 so the correct answer of 4 needs to go in the box called 4. But the box called 4 already has a 3 in it, so our initial choice of numbers is a bad one.
Can you do better?
It is very difficult to come up with the solution from scratch (but do try!). Here are a few numbers already filled in, if you would like a starting point (and as a pdf).
The solution is not unique, but here is Ludgate’s solution (and as a pdf).
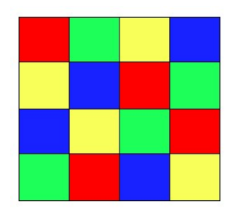
In a Latin Square each symbol or colour occurs exactly once in each row and exactly once in each column.
Can you complete this one? It is from Brilliant.org where you can do it interactively.
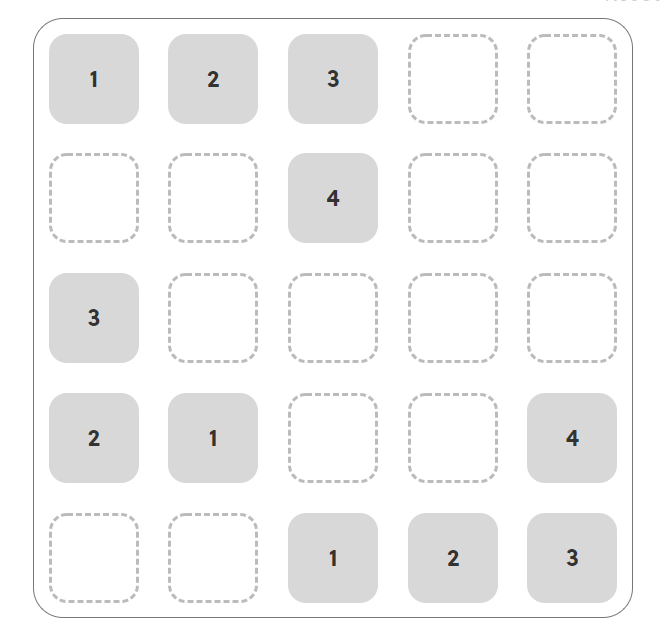
Now for the hard challenge …

Thanks to NRich for this great puzzle
Today we were inspired by this amazing video from 3 Blue 1 Brown.
Can you always draw a rectangle on any shape? How about a square?
Here is a series of challenges on paper and geogebra to explore this fascinating topic.

Here are the 1st and 2nd explorations we did today to learn about modular arithmetic and a fantastic geogebra app.