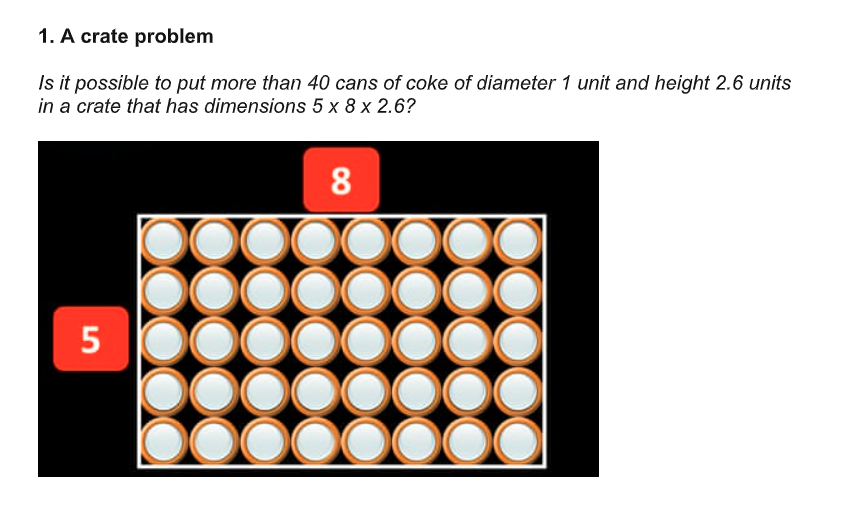
We solved puzzles today inspired by the work of Fields Medalists chosen by Alex Bellos.

We solved puzzles today inspired by the work of Fields Medalists chosen by Alex Bellos.
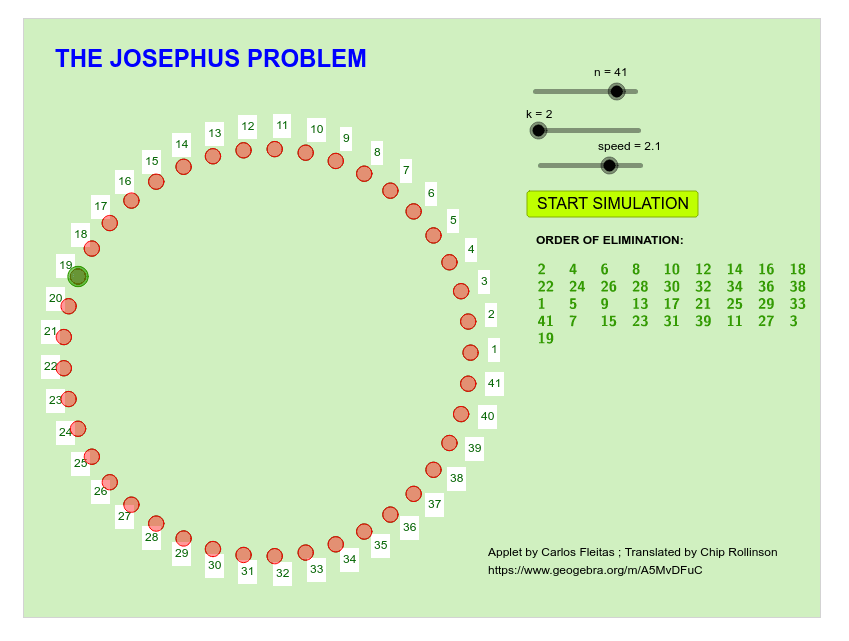
This week we solved a tricky puzzle involving swords and trying to sit in the right position of a circle to survive!
A powerpoint, created at the Ghana Maths Camp in 2015, explaining the puzzle and with solution is here.
We looked at some fun card tricks with maths behind them.

This highly addictive shape game is brilliant fun and has some interesting mathematical ideas to explore. We started with drawing all the one block, two block, three block and four block shapes we could think of. No repeats (reflections, rotations) were allowed – for example these two shapes of four blocks are effectively the same:


There are 9 different shapes, and the game contains each of these possibilities. There are 7 dice which you throw to generate where to put 7 wooden pieces. For example the game could start like this:
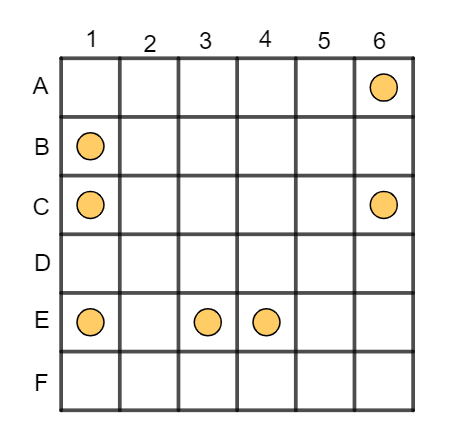
The values on the dice are:

Once you have the wooden pieces in place, it is a two player race to fit all the coloured pieces on the grid. Given the dice configuration above, how many possible games are there to play? The makers of the game have ensured all these games are possible in at least one way.
After we played a few times, we tried to make up an impossible configuration of wooden pieces (ignoring the dice). Here is a trivially impossible one (because there is only one blue one block piece):

But can you make up one that looks like it could work but then doesn’t? Ghazi even found a way of generating impossible solutions and proving they were impossible.

Puzzle 1 – The classic
You are on one side of a river, and with you, there is a wolf, a goat and a cabbage. You have one boat, and can only take one living thing at a time. The goat cannot be left alone with the cabbage and the wolf cannot be left alone with the goat. How many journeys must you do in minimum to get all the objects to the other side of the river? In how many different ways can you do it?
See this video for a brilliant extension leading to an explanation of Vertex Cover.
Puzzle 2 – The answer is smaller than you think …
There are 4 people (A, B, C and D) who want to cross a bridge in night.
There is only one torch with them and the bridge cannot be crossed without the torch. There cannot be more than two persons on the bridge at any time, and when two people cross the bridge together, they must move at the slower person’s pace.
What is the quickest way for all four to cross the bridge?
Puzzle 3 – Lions and Wildebeests
3 lions and 3 wildebeests need to cross a river using a raft.
The raft needs at least one animal to paddle it across the river, and it can hold at most two animals.
If the lions ever outnumber the wildebeest on either side of the river (including the animals in the boat it’s on that side) they will eat the wildebeest.
The animals can’t just swim across.
How many crossings does it take to get the animals across the river?
For the solution see this nice video.
A great game from NRich.
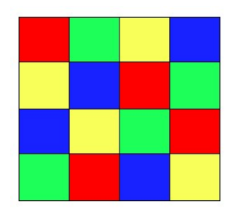
In a Latin Square each symbol or colour occurs exactly once in each row and exactly once in each column.
Can you complete this one? It is from Brilliant.org where you can do it interactively.
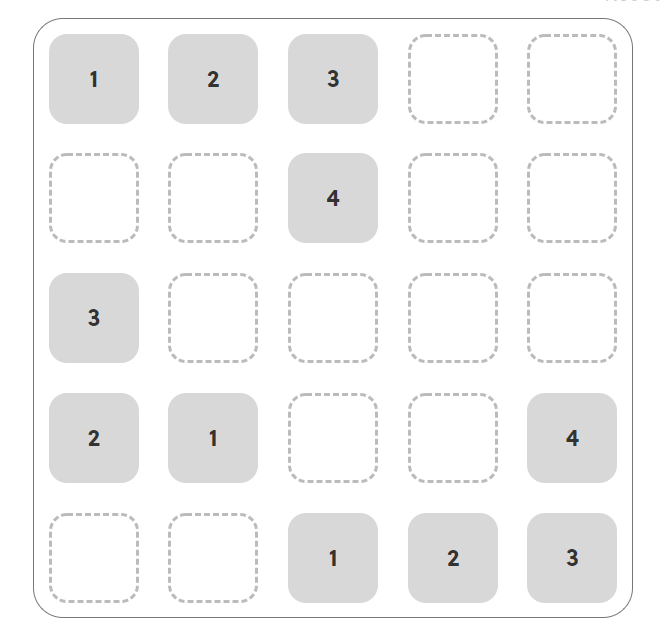
Now for the hard challenge …

Thanks to NRich for this great puzzle

Today we tried to get an insight into qubits – which can be 0,1 or a superposition of both simultaneously. Complex problems can theoretically be solved much faster using qubits instead of bits.
Terry Rudolph has written an excellent book suitable for high school students on this subject. We went through a few excerpts collated here, and then looked at pages 27 – 31 of Part 1 of his book.

Today we solved the first three puzzles from The Alan Turing Cryptography Competition 2022. You can see a sample archived competition here.