On the 10th of October we engaged in an activity in which thousands of students around the world were concurrently engaged.
First, pick a rectangle in the room (e.g. table, computer screen, piece of paper). Take a piece of string and make it the length of the perimeter of the rectangle plus one metre.
Now try and hold the string around the shape so that you have made a path of equal width around the shape. How wide is your path?
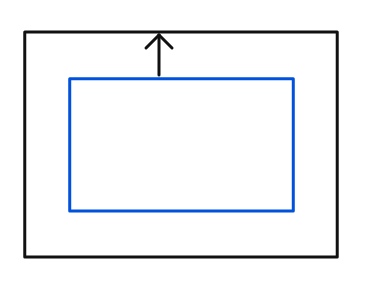
We did this practically and then resorted to pen and paper to find exact widths for different sized starting rectangles.
Amazingly we all found the same width …
We then tried the same puzzle but keeping the path the same width all the way round the shape:
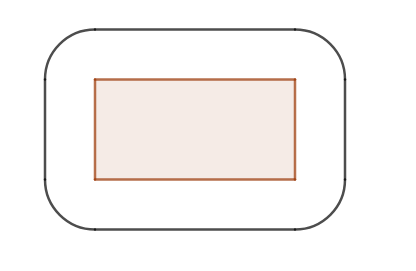
Finally we tried with a circle
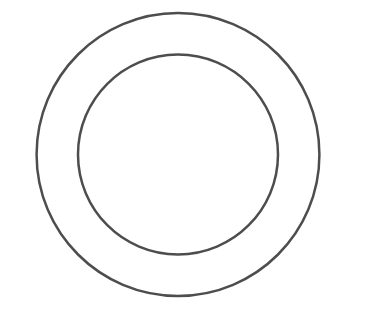
which led to us being able to understand the answer to this classic puzzle:
Imagine that the Earth is a perfect sphere and imagine we have a large piece of string tied around the equator.
We then add one meter to the length of the string so that now the string is hovering above the equator, still in a circle.
What is the size of the gap between the equator and the piece of string? Enough to fit a piece of paper under? A cat? A car?
For the solution see here.
We prepared for this session online with our maths club colleagues in Kenya and here is their brilliant report of their session on the same day.